Isotropic


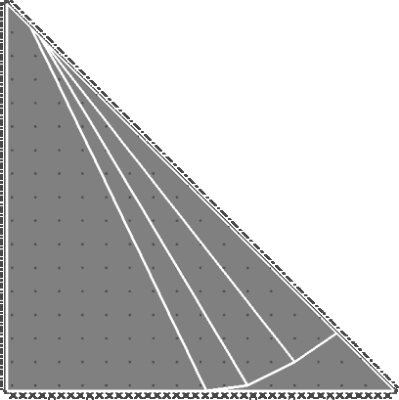
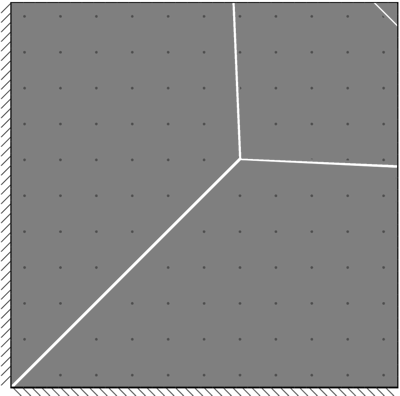
Square with fixed supports, modelled with eighth symmetry

Benchmark 42.85
Result 43.04
Discrepancy on Collapse Load   0.43%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One fixed edge and two lines of symmetry

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

42.851

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998), p 506. Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Square with fixed supports

Benchmark 42.85
Result 43.26
Discrepancy on Collapse Load   0.94%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Four fixed edges

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

42.851

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998), p 506. Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Irregular hexagon with simple supports, modelled with quarter symmetry

Benchmark 5.5
Result 5.5
Discrepancy on Collapse Load   0.02%

General Description

Hexagonal reinforced concrete slab

Key Dimensions

a = 1.414m, b = 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

5.5

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998), p 504. Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Irregular hexagon with simple supports

Benchmark 5.5
Result 5.5
Discrepancy on Collapse Load   0.02%

General Description

Hexagonal reinforced concrete slab

Key Dimensions

a = 1.414m, b = 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Six simply supported edges

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

5.5

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998), p 504. Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Square with simple supports, modelled with eighth symmetry

Benchmark 24
Result 24
Discrepancy on Collapse Load   0%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Four simply supported edges

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

24

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998), p 504. Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Square with simple supports

Benchmark 24
Result 24
Discrepancy on Collapse Load   0%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Four simply supported edges

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

24

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998), p 506. Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Square with three simply supported edges

Benchmark 14.14
Result 14.16
Discrepancy on Collapse Load   0.14%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Three simply supported edges and one free edge

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

14.14

Reference

J. Wüst and W. Wagner, Systematic prediction of yield-line configurations for arbitrary polygonal plates, Engineering Structures 30 (2008), pp. 2081-2093. Available from http://dx.doi.org/10.1016/j.engstruct.2008.01.005



Triangle with two simply supported edges, mp = 100kNm/m

Benchmark 12
Result 12.01
Discrepancy on Collapse Load   0.09%

General Description

Triangular reinforced concrete slab

Key Dimensions

10m x 10m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two simply supported edges and one free edge

Concrete Properties

m = 100kNm/m

Partial Factors

Unity

Benchmark Solution

12

Reference

A. Thavalingam, A. Jennings, D. Sloan and J.J. McKeown, Computer-assisted generation of yield-line patterns for uniformly loaded isotropic slabs using an optimisation strategy, Engineering Structures 21 (1999), pp. 488-496. Available from http://dx.doi.org/10.1016/S0141-0296(97)00228-9



Rectangle with indent, with three fixed edges, two simply supported edges and one free edge, mp = 100kNm/m

Benchmark 29.2
Result 29.17
Discrepancy on Collapse Load   -0.1%

General Description

Rectangular reinforced concrete slab with indent

Key Dimensions

6m x 10m, indent 2.5m x 1.5m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Fixed edges around indent and on adjoining edge, simply supported on two opposite short edges and free on one long edge

Concrete Properties

m = 100kNm/m

Partial Factors

Unity

Benchmark Solution

29.2

Reference

A. Thavalingam, A. Jennings, D. Sloan and J.J. McKeown, Computer-assisted generation of yield-line patterns for uniformly loaded isotropic slabs using an optimisation strategy, Engineering Structures 21 (1999), pp. 488-496. Available from http://dx.doi.org/10.1016/S0141-0296(97)00228-9



Rectangle with two alcoves, with seven fixed edges, mp = 100kNm/m

Benchmark 35.8
Result 35.79
Discrepancy on Collapse Load   -0.03%

General Description

Rectangular reinforced concrete slab with two alcoves

Key Dimensions

6m x 10m, alcoves 2m x 2m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Fixed on all edges except long edge facing alcoves

Concrete Properties

m = 100kNm/m

Partial Factors

Unity

Benchmark Solution

35.8

Reference

A. Thavalingam, A. Jennings, D. Sloan and J.J. McKeown, Computer-assisted generation of yield-line patterns for uniformly loaded isotropic slabs using an optimisation strategy, Engineering Structures 21 (1999), pp. 488-496. Available from http://dx.doi.org/10.1016/S0141-0296(97)00228-9



Trapezium with three simply supported edges, modelled with symmetry

Benchmark 0.28
Result 0.28
Discrepancy on Collapse Load   1.54%

General Description

Trapezoidal reinforced concrete slab

Key Dimensions

Long edge 10m, width 10m, short edge 5m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Simply supported on three edges and free on fourth edge modelled with one line of symmetry

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

0.28

Reference

A. Thavalingam, A. Jennings, D. Sloan and J.J. McKeown, Computer-assisted generation of yield-line patterns for uniformly loaded isotropic slabs using an optimisation strategy, Engineering Structures 21 (1999), pp. 488-496. Available from http://dx.doi.org/10.1016/S0141-0296(97)00228-9



L-shaped with two simply supported edges and four free edges, mp = 11.72kNm/m

Benchmark 10
Result 10.03
Discrepancy on Collapse Load   0.31%

General Description

L-shaped reinforced concrete slab

Key Dimensions

2.5m x 1.2m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Simply supported on opposite ends

Concrete Properties

m = 11.72kNm/m

Partial Factors

Unity

Benchmark Solution

10

Reference

A.C.A. Ramsay and D. Johnson, Analysis of practical slab configurations using automated yield-line analysis and geometric optimization of fracture patterns, Engineering Structures 20 (1998), pp. 647-654. Available from http://dx.doi.org/10.1016/S0141-0296(97)00111-9



Rectangle supported as a propped cantilever, mp = 5kNm/m

Benchmark 14.57
Result 14.57
Discrepancy on Collapse Load   0.01%

General Description

Rectangular reinforced concrete slab

Key Dimensions

2m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Fixed and simply supported on opposite short edges

Concrete Properties

m = 5kNm/m

Partial Factors

Unity

Benchmark Solution

14.57

Reference

A.C.A. Ramsay and D. Johnson, Geometric optimization of yield-line patterns using a direct search method, Structural and Multidisciplinary Optimization 14 (1997), pp. 108-115. Available from http://www.springerlink.com/content/g022p37175707016/



Square with three simply supported edges

Benchmark 0.14
Result 0.14
Discrepancy on Collapse Load   1.13%

General Description

Square reinforced concrete slab

Key Dimensions

10m x 10m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Simply supported on three edges and free on fourth edge

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

0.14

Reference

A.C.A. Ramsay and D. Johnson, Geometric optimization of yield-line patterns using a direct search method, Structural and Multidisciplinary Optimization 14 (1997), pp. 108-115. Available from http://www.springerlink.com/content/g022p37175707016/



Square with fixed supports, modelled with eighth symmetry

Benchmark 2.68
Result 2.69
Discrepancy on Collapse Load   0.37%

General Description

Square reinforced concrete slab

Key Dimensions

4m x 4m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One fixed edge and two lines of symmetry

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

2.68

Reference

S. Krenk, L. Damkilde and O. Høyer, Limit Analysis and Optimal Design of Plates with Equilibrium Elements, Journal of Engineering Mechanics 120 (1994), pp. 1237-1254. Available from http://dx.doi.org/10.1061/(ASCE)0733-9399(1994)120:6(1237)



Hexagon with simple supports, modelled with sixth symmetry

Benchmark 6
Result 6
Discrepancy on Collapse Load   0%

General Description

Hexagonal reinforced concrete slab

Key Dimensions

h = 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

6

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998). Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Trapezium with three simply supported edges

Benchmark 0.285
Result 0.285
Discrepancy on Collapse Load   0%

General Description

Trapezoidal reinforced concrete slab

Key Dimensions

Long edge 10m, width 10m, short edge 5m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Three simply supported edges and one free edge

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

0.285

Reference

A.K.H. Kwan, Dip and strike angles method for yield line analysis of reinforced concrete slabs, Magazine of Concrete Research 56 (2004), pp. 487-498. Available from http://www.atypon-link.com/TELF/doi/pdf/10.1680/macr.56.8.487.49450



Square with two simply supported edges and one column

Benchmark 10.67
Result 10.261
Discrepancy on Collapse Load   -3.98%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two adjacent simply supported edges with column at corner of free edges (column as simple support from x/y = 0.9999 to x/y = 1.0)

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

10.67

Reference

A.K.H. Kwan, Dip and strike angles method for yield line analysis of reinforced concrete slabs, Magazine of Concrete Research 56 (2004), pp. 487-498. Available from http://www.atypon-link.com/TELF/doi/pdf/10.1680/macr.56.8.487.49450



Square with one simply supported edge and one column

Benchmark 5.17
Result 4.055
Discrepancy on Collapse Load   -21.57%
Note   It is evident that the failure mechanism identified using LimitState:SLAB (adjacent to the column) is different to that proposed by Kwan (single yield-line diagonally across the center of the slab). This is the reasoning for the discrepancy in collapse load obtained.

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and one column at corner of two free edges (column as simple support over distance of 0.0001m in x and y directions)

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

5.17

Reference

A.K.H. Kwan, Dip and strike angles method for yield line analysis of reinforced concrete slabs, Magazine of Concrete Research 56 (2004), pp. 487-498. Available from http://www.atypon-link.com/TELF/doi/pdf/10.1680/macr.56.8.487.49450



Irregular polygon with two fixed edges and two columns

Benchmark 0.1967
Result 0.1865
Discrepancy on Collapse Load   -5.2%

General Description

Polygonal reinforced concrete slab

Key Dimensions

12m x 10m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two adjacent fixed edges with two columns at corners of free edges (columns as simple support over distance of < 0.0001m in x and y directions)

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

0.1967

Reference

A.K.H. Kwan, Dip and strike angles method for yield line analysis of reinforced concrete slabs, Magazine of Concrete Research 56 (2004), pp. 487-498. Available from http://www.atypon-link.com/TELF/doi/pdf/10.1680/macr.56.8.487.49450



45 degree triangle with two simply supported edges

Benchmark 35.3
Result 35.53
Discrepancy on Collapse Load   0.65%

General Description

Triangular reinforced concrete slab

Key Dimensions

Side lengths 1m, angle 45 degrees

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two simply supported edges and one free edge

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

35.3

Reference

D. Johnson, Yield-line analysis by sequential linear programming, International Journal of Solids and Structures 32 (1995), pp. 1395-1404. Available from http://dx.doi.org/10.1016/0020-7683(94)00200-G



90 degree triangle with two simply supported edges

Benchmark 12
Result 12
Discrepancy on Collapse Load   0%

General Description

Triangular reinforced concrete slab

Key Dimensions

Side lengths 1m, angle 90 degrees

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two simply supported edges and one free edge

Concrete Properties

m = 1kNm/m

Partial Factors

Unity

Benchmark Solution

12

Reference

D. Johnson, Yield-line analysis by sequential linear programming, International Journal of Solids and Structures 32 (1995), pp. 1395-1404. Available from http://dx.doi.org/10.1016/0020-7683(94)00200-G


Orthotropic


Octagon with simple supports, orthotropically reinforced with m = 5.83kNm/m, m' = 1kNm/m, modelled with eighth symmetry

Benchmark 34.97
Result 34.97
Discrepancy on Collapse Load   0%

General Description

Octagonal reinforced concrete slab

Key Dimensions

h = 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 5.83kNm/m, m' = 1kNm/m

Partial Factors

Unity

Benchmark Solution

34.971

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998), p 506. Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Irregular hexagon (a) with simple supports, orthotropically reinforced with m = 1kNm/m, m' = 0kNm/m

Benchmark 17.75
Result 16.09
Discrepancy on Collapse Load   -9.34%

General Description

Hexagonal reinforced concrete slab

Key Dimensions

1.5m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Six simply supported edges

Concrete Properties

m = 1kNm/m, m' = 0kNm/m

Partial Factors

Unity

Benchmark Solution

17.75

Reference

J. Wüst and W. Wagner, Systematic prediction of yield-line configurations for arbitrary polygonal plates, Engineering Structures 30 (2008), pp. 2081-2093. Available from http://dx.doi.org/10.1016/j.engstruct.2008.01.005



Irregular hexagon (b) with simple supports, orthotropically reinforced with m = 1kNm/m, m' = 0kNm/m

Benchmark 54.4
Result 48.42
Discrepancy on Collapse Load   -10.99%

General Description

Hexagonal reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Six simply supported edges

Concrete Properties

m = 1kNm/m, m' = 0kNm/m

Partial Factors

Unity

Benchmark Solution

54.4

Reference

J. Wüst and W. Wagner, Systematic prediction of yield-line configurations for arbitrary polygonal plates, Engineering Structures 30 (2008), pp. 2081-2093. Available from
http://dx.doi.org/10.1016/j.engstruct.2008.01.005



Pentagon with simple supoorts, orthotropically reinforced with m = 1.89kNm/m, m' = 1kNm/m, modelled with fifth symmetry

Benchmark 11.37
Result 11.37
Discrepancy on Collapse Load   0.03%

General Description

Pentagonal reinforced concrete slab

Key Dimensions

h = 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 1.89kNm/m, m' = 1kNm/m

Partial Factors

Unity

Benchmark Solution

11.367

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998). Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Septagon with simple supports, orthotropically reinforced with m = 4.31kNm/m, m' = 1kNm/m, modelled with seventh symmetry

Benchmark 25.87
Result 25.87
Discrepancy on Collapse Load   0.01%

General Description

Septagonal reinforced concrete slab

Key Dimensions

h = 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 4.31kNm/m, m' = 1kNm/m

Partial Factors

Unity

Benchmark Solution

25.872

Reference

M.P. Nielsen, Limit analysis and concrete plasticity, CRC Press (1998). Available from http://books.google.co.uk/books?id=HNJNwkDlI3kC&lpg=PP1&pg=PP1



Rectangle with three simply supported edges, orthotropically reinforced with mx = 0.4kNm/m, my = 1kNm/m

Benchmark 19.06
Result 19.06
Discrepancy on Collapse Load   0%

General Description

Rectangular reinforced concrete slab

Key Dimensions

1m x 0.4m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Three simply supported edges and one free edge

Concrete Properties

mx = m'x = 0.4kNm/m, my = m'y = 1kNm/m

Partial Factors

Unity

Benchmark Solution

19.06

Reference

A.K.H. Kwan, Dip and strike angles method for yield line analysis of reinforced concrete slabs, Magazine of Concrete Research 56 (2004), pp. 487-498. Available from http://www.atypon-link.com/TELF/doi/pdf/10.1680/macr.56.8.487.49450



Rectangle with three simply supported edge and one fixed edge, orthotropically reinforced with mx = 1kNm/m, my = 0.3kNm/m

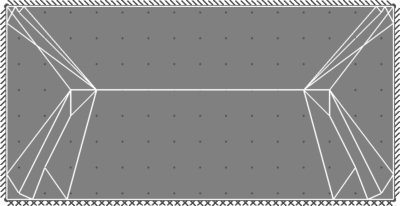
Benchmark 0.15
Result 0.15
Discrepancy on Collapse Load   0.48%

General Description

Rectangular reinforced concrete slab

Key Dimensions

20m x 10m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Three simply supported edges and one fixed edge

Concrete Properties

mx = m'x = 1kNm/m, my = m'y = 0.3kNm/m

Partial Factors

Unity

Benchmark Solution

0.151

Reference

A.K.H. Kwan, Dip and strike angles method for yield line analysis of reinforced concrete slabs, Magazine of Concrete Research 56 (2004), pp. 487-498. Available from http://www.atypon-link.com/TELF/doi/pdf/10.1680/macr.56.8.487.49450



Triangle with two simply supported edges, orthotropically reinforced with m = 100kNm/m, m' = 50kNm/m

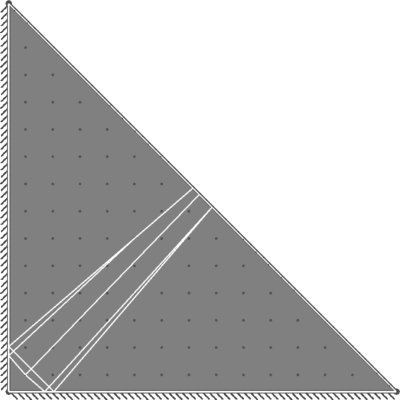
Benchmark 11.72
Result 11.66
Discrepancy on Collapse Load   -0.53%

General Description

Triangular reinforced concrete slab

Key Dimensions

10m x 10m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two simply supported edges and one free edge

Concrete Properties

m = 100kNm/m, m' = 50kNm/m

Partial Factors

Unity

Benchmark Solution

11.72

Reference

A. Thavalingam, A. Jennings, D. Sloan and J.J. McKeown, Computer-assisted generation of yield-line patterns for uniformly loaded isotropic slabs using an optimisation strategy, Engineering Structures 21 (1999), pp. 488-496. Available from http://dx.doi.org/10.1016/S0141-0296(97)00228-9



Triangle with two simply supported edges, orthotropically reinforced with m = 100kNm/m, m' = 0kNm/m

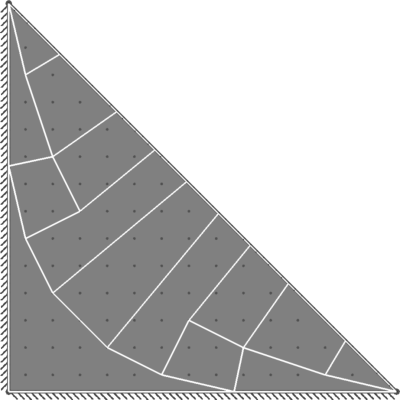
Benchmark 9.5
Result 9.43
Discrepancy on Collapse Load   -0.76%

General Description

Triangular reinforced concrete slab

Key Dimensions

10m x 10m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two simply supported edges and one free edge

Concrete Properties

m = 100kNm/m, m' = 0kNm/m

Partial Factors

Unity

Benchmark Solution

9.5

Reference

A. Thavalingam, A. Jennings, D. Sloan and J.J. McKeown, Computer-assisted generation of yield-line patterns for uniformly loaded isotropic slabs using an optimisation strategy, Engineering Structures 21 (1999), pp. 488-496. Available from http://dx.doi.org/10.1016/S0141-0296(97)00228-9



Rectangle with fixed supports, orthotropically reinforced with mx = 2kNm/m, my = 1kNm/m, modelled with quarter symmetry

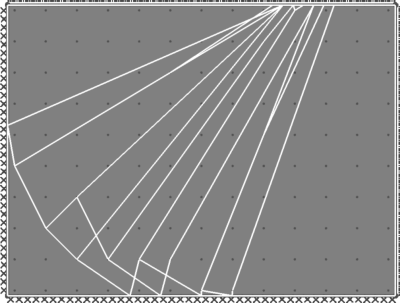
Benchmark 104
Result 94.44
Discrepancy on Collapse Load   -9.19%

General Description

Rectangular reinforced concrete slab

Key Dimensions

1m x 0.75m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two fixed edges and two lines of symmetry

Concrete Properties

mx = m'x = 2kNm/m, my = m'y = 1kNm/m

Partial Factors

Unity

Benchmark Solution

104

Reference

D. Johnson, Automated yield-line analysis of orthotropic slabs, International Journal of Solids and Structures 33 (1996), pp. 1-10. Available from http://dx.doi.org/10.1016/0020-7683(95)00025-6



Rectangle with three simply supported edges, orthotropically reinforced with mx = 1kNm/m, my = 2kNm/m

Benchmark 8.87
Result 8.84
Discrepancy on Collapse Load   -0.37%

General Description

Rectangular reinforced concrete slab

Key Dimensions

2m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Three simply supported edges and one free edge

Concrete Properties

mx = m'x = 2kNm/m, my = m'y = 1kNm/m

Partial Factors

Unity

Benchmark Solution

8.87

Reference

D. Johnson, Yield-line analysis by sequential linear programming, International Journal of Solids and Structures 32 (1995), pp. 1395-1404. Available from http://dx.doi.org/10.1016/0020-7683(94)00200-G



Rectangle with one fixed edge and two columns, orthotropically reinforced with m = 1kNm/m, m' = 1.5kNm/m

Benchmark 3.33
Result 3.33
Discrepancy on Collapse Load   0.04%

General Description

Rectangular reinforced concrete slab

Key Dimensions

1m x 2m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One short fixed edge with one column on opposite edge and one line of symmetry

Concrete Properties

m = 1kNm/m, m' = 1.5kNm/m

Partial Factors

Unity

Benchmark Solution

3.333

Reference

D. Johnson, Yield-line analysis by sequential linear programming, International Journal of Solids and Structures 32 (1995), pp. 1395-1404. Available from http://dx.doi.org/10.1016/0020-7683(94)00200-G



45 degree triangle with two simply supported edges, orthotropically reinforced with m = 1kNm/m, m' = 0kNm/m

Benchmark 30.1
Result 28.55
Discrepancy on Collapse Load   -5.14%

General Description

Triangular reinforced concrete slab

Key Dimensions

Side lengths 1m, angle 45 degrees

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two simply supported edges and one free edge

Concrete Properties

m = 1kNm/m, m' = 0kNm/m

Partial Factors

Unity

Benchmark Solution

30.1

Reference

D. Johnson, Yield-line analysis by sequential linear programming, International Journal of Solids and Structures 32 (1995), pp. 1395-1404. Available from http://dx.doi.org/10.1016/0020-7683(94)00200-G



45 degree triangle with two simply supported edges, orthotropically reinforced with m = 1kNm/m, m' = 0.5kNm/m

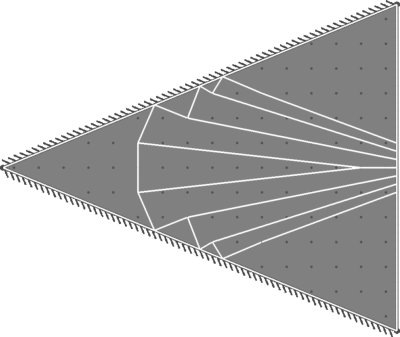
Benchmark 34.03
Result 33.15
Discrepancy on Collapse Load   -2.6%

General Description

Triangular reinforced concrete slab

Key Dimensions

Side lengths 1m, angle 45 degrees

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two simply supported edges and one free edge

Concrete Properties

m = 1kNm/m, m' = 0.5kNm/m

Partial Factors

Unity

Benchmark Solution

34.03

Reference

D. Johnson, Yield-line analysis by sequential linear programming, International Journal of Solids and Structures 32 (1995), pp. 1395-1404. Available from http://dx.doi.org/10.1016/0020-7683(94)00200-G



90 degree triangle with two simply supported edges, orthotropically reinforced with m = 1kNm/m, m' = 0kNm/m

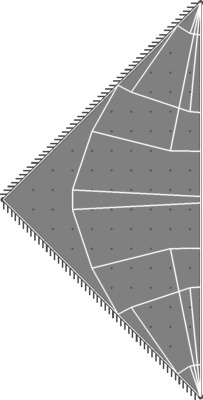
Benchmark 10.17
Result 9.5
Discrepancy on Collapse Load   -6.57%

General Description

Triangular reinforced concrete slab

Key Dimensions

Side lengths 1m, angle 90 degrees

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two simply supported edges and one free edge

Concrete Properties

m = 1kNm/m, m' = 0kNm/m

Partial Factors

Unity

Benchmark Solution

10.17

Reference

D. Johnson, Yield-line analysis by sequential linear programming, International Journal of Solids and Structures 32 (1995), pp. 1395-1404. Available from http://dx.doi.org/10.1016/0020-7683(94)00200-G



90 degree triangle with two simply supported edges, orthotropically reinforced with m = 1kNm/m, m' = 0kNm/m

Benchmark 11.7
Result 11.66
Discrepancy on Collapse Load   -0.36%

General Description

Triangular reinforced concrete slab

Key Dimensions

Side lengths 1m, angle 90 degrees

Adequacy Factor on Load

Unit area load

Boundary Conditions

Two simply supported edges and one free edge

Concrete Properties

m = 1kNm/m, m' = 0.5kNm/m

Partial Factors

Unity

Benchmark Solution

11.7

Reference

D. Johnson, Yield-line analysis by sequential linear programming, International Journal of Solids and Structures 32 (1995), pp. 1395-1404. Available from http://dx.doi.org/10.1016/0020-7683(94)00200-G



Square with simple supports, orthotropically reinforced with m = 1kNm/m, m' = 0.5kNm/m, modelled with eighth symmetry

Benchmark 24
Result 23.56
Discrepancy on Collapse Load   -1.82%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 1kNm/m, m' = 0.5kNm/m

Partial Factors

Unity

Benchmark Solution

23.262

Reference

S. Krenk, L. Damkilde and O. Høyer, Limit Analysis and Optimal Design of Plates with Equilibrium Elements, Journal of Engineering Mechanics 120 (1994), pp. 1237-1254. Available from http://dx.doi.org/10.1061/(ASCE)0733-9399(1994)120:6(1237)



Square with simple supports, orthotropically reinforced with m = 1kNm/m, m' = 0.333kNm/m, modelled with eighth symmetry

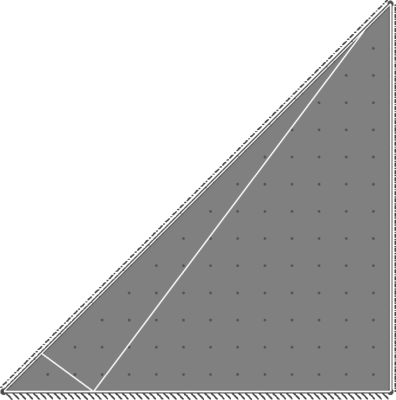
Benchmark 23.26
Result 23.17
Discrepancy on Collapse Load   -0.38%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 1kNm/m, m' = 0.333kNm/m

Partial Factors

Unity

Benchmark Solution

22.801

Reference

S. Krenk, L. Damkilde and O. Høyer, Limit Analysis and Optimal Design of Plates with Equilibrium Elements, Journal of Engineering Mechanics 120 (1994), pp. 1237-1254. Available from http://dx.doi.org/10.1061/(ASCE)0733-9399(1994)120:6(1237)



Square with simple supports, orthotropically reinforced with m = 1kNm/m, m' = 0.25kNm/m, modelled with eighth symmetry

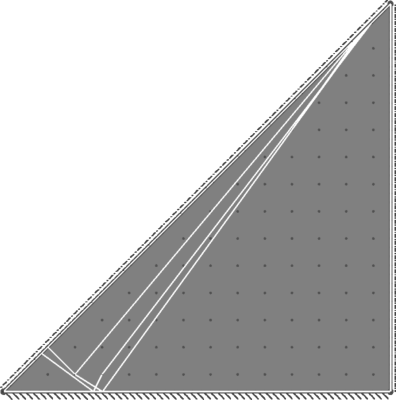
Benchmark 22.8
Result 22.89
Discrepancy on Collapse Load   0.38%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 1kNm/m, m' = 0.25kNm/m

Partial Factors

Unity

Benchmark Solution

22.419

Reference

S. Krenk, L. Damkilde and O. Høyer, Limit Analysis and Optimal Design of Plates with Equilibrium Elements, Journal of Engineering Mechanics 120 (1994), pp. 1237-1254. Available from http://dx.doi.org/10.1061/(ASCE)0733-9399(1994)120:6(1237)



Square with simple supports, orthotropically reinforced with m = 1kNm/m, m' = 0.125kNm/m, modelled with eighth symmetry

Benchmark 22.42
Result 22.33
Discrepancy on Collapse Load   -0.41%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 1kNm/m, m' = 0.125kNm/m

Partial Factors

Unity

Benchmark Solution

21.878

Reference

S. Krenk, L. Damkilde and O. Høyer, Limit Analysis and Optimal Design of Plates with Equilibrium Elements, Journal of Engineering Mechanics 120 (1994), pp. 1237-1254. Available from http://dx.doi.org/10.1061/(ASCE)0733-9399(1994)120:6(1237)



Square with simple supports, orthotropically reinforced with m = 1kNm/m, m' = 0kNm/m, modelled with eighth symmetry

Benchmark 22
Result 21.53
Discrepancy on Collapse Load   -2.14%

General Description

Square reinforced concrete slab

Key Dimensions

1m x 1m

Adequacy Factor on Load

Unit area load

Boundary Conditions

One simply supported edge and two lines of symmetry

Concrete Properties

m = 1kNm/m, m' = 0kNm/m

Partial Factors

Unity

Benchmark Solution

22

Reference

S. Krenk, L. Damkilde and O. Høyer, Limit Analysis and Optimal Design of Plates with Equilibrium Elements, Journal of Engineering Mechanics 120 (1994), pp. 1237-1254. Available from http://dx.doi.org/10.1061/(ASCE)0733-9399(1994)120:6(1237)



Trapezium with three simply supported edges, orthotropically reinforced with mx = 1kNm/m, my = 2kNm/m, m' = 0kNm/m

Benchmark 8.45
Result 7.28
Discrepancy on Collapse Load   -13.9%

General Description

Trapezoidal reinforced concrete slab

Key Dimensions

Long edge 20m, height 20m, short edge 10m

Adequacy Factor on Load

Unit area load

Boundary Conditions

Three simply supported edges and one free edge

Concrete Properties

mx = 1kNm/m, my = 2kNm/m, m'x = m'y = 0kNm/m

Partial Factors

Unity

Benchmark Solution

8.446

Reference

K.V. Balasubramanyam and V. Kalyanaraman, Yield-Line Analysis by Linear Programming, Journal of Structural Engineering 114 (1988), pp. 1431-1437. Available from http://dx.doi.org/10.1061/(ASCE)0733-9445(1988)114:6(1431)